Calculate the Volume of a Prism

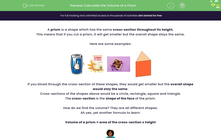
A prism is a shape which has the same cross-section throughout its height.

This means that if you cut a prism, it will get smaller but the overall shape stays the same.

Here are some examples:

I      

If you sliced through the cross-section of these shapes, they would get smaller but the overall shape would stay the same.

Cross-sections of the shapes above would be a circle, rectangle, square and triangle.

The cross-section is the shape of the face of the prism.

How do we find the volume? They are all different shapes.

Ah yes, yet another formula to learn:

Volume of a prism = area of the cross-section x height

 Before we continue, we need a recap of how to find the area of these shapes.

Area of a square = base x height

Area of a rectangle = base x height

Area of a triangle = base x height ÷ 2

Area of a circle =  π x r x r

Can you remember the value of π?  Don't worry, you will find out later if you have forgotten.

Almost there.  To find the volume we calculate the area of the face first and then multiply by the height.

 Time to practise.

Find the volume of this box of chocolates. Base = 6 cm

The face (cross-section) is a square - find the area first.

 6 x 6 = 36 cm, now just multiply by the length which, as it's a square box , is also 6 cm.   36 x 6 = 216 cm³

Find the volume of the cornflakes box.  Base 12 cm, height 6 cm, length 30 cm

The face (cross-section) is a rectangle - find the area first. 

12 x 6 = 72 cm, now just multiply by the length   72 x 30 = 2160 cm³

Find the volume of the triangular prism.  Base 4 cm, height 4 cm, length 12 cm

The face (cross-section) is a triangle - find the area first. 

4 x 4 = 16 ÷ 2 = 8 cm, now just multiply by the length 8 x 12 = 96 cm³

Find the volume of this can.  Radius 4 cm, length 8 cm

The face (cross-section) is a circle - find the area first. 

Did you remember the value of π? Of course,  it's 3.142

Area = 3.142 x 4 x 4 = 50.27

Now multiply by the length 50.27 x 8 = 402.16 cm³

That was a bit of a marathon, wasn't it?  Guess what, it is not over...

Activity time!