Recognise Parallel and Perpendicular Lines From Their Gradients

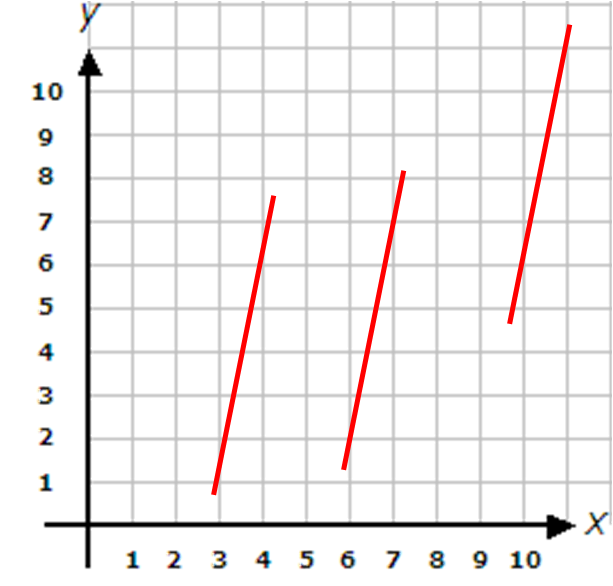
Parallel lines have the same gradient.

All of these lines have a gradient of 5.

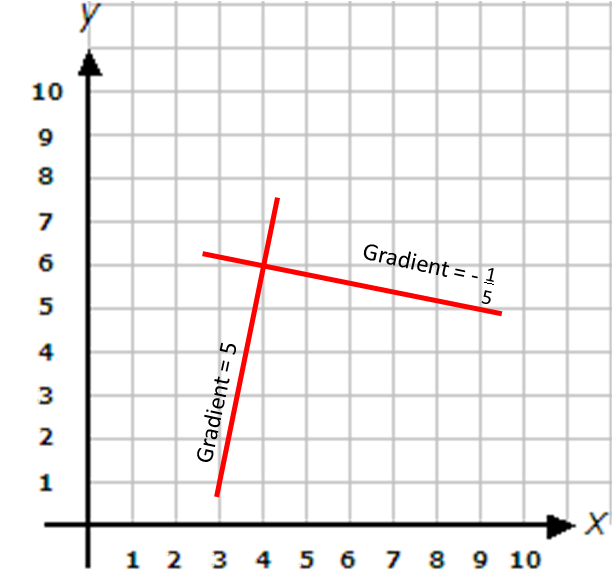
Perpendicular lines have gradients which multiply together to give -1.

Here, one line has a gradient of 5 and the other of -1/5.

To find the gradient of a line which is perpendicular to one of gradient a/b, we invert the fraction and multiply by -1.

This means you don't need diagrams to check if lines are perpendicular.

Example

State the gradient of a line which is perpendicular to one of gradient ¾.

Answer

Invert the fraction and multiply by -1.