Find a Missing Angle Using the Tan Ratio

You may have used a "SOH CAH TOA" formula triangle to find a missing side of a right-angled triangle.

You can also use them to find the missing angle in a right-angled triangle.

Here, we are going to look at the tan formula triangle and how to calculate an angle from it.

Let's remind ourselves of the formula triangle:

T=O/A

T = tan (of angle)

A = adjacent length

O = opposite length

The formula is T = O/A. This means:

tan(angle) = O/A

You may have used formula this to find an opposite or adjacent length when you had an angle.

If you are finding an angle, you substitute the lengths into the same formula - you just need to then use a function known as inverse tan to calculate it (this is written as tan^-1). On most calculators, this is accessed by pressing SHIFT and then tan.

So, the formula for finding an angle with an adjacent and opposite is:

angle = tan^-1(O/A)

Let's give it a go...

IMPORTANT NOTE: Make sure your calculator is set to degrees. You can tell as a "D" appears at the top of the calculator screen.

Example 

Find angle x in this triangle.

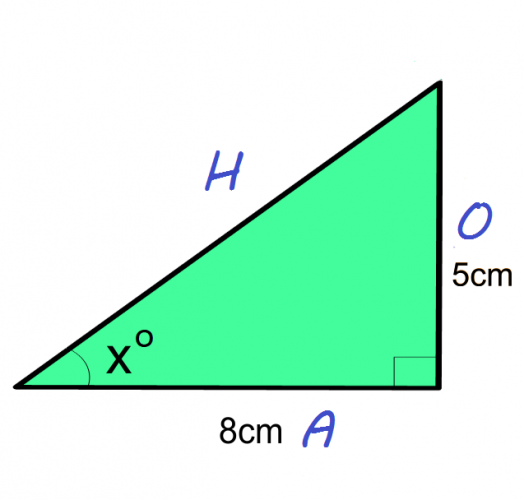
1. Label the triangle. 

2. Identify the two sides you want to use. If you want to find the angle using tan, you will need the opposite, O, and adjacent, A.

3. Substitute the lengths into the formula T = O ÷ A to calculate tan x.

5 ÷ 8 = 0.625

tan x = 0.625

4. Finally, use the inverse tan button (tan^-1) on your calculator on this value.

x = tan^-1(0.625) = 32.0° (1 d.p.)

If you follow these steps, you should be able to find the angle in a right angled triangle when you have A and O.

Have a go at this activity and practice this skill!