Find a Missing Angle Using the Sin Rule

Did you know that trigonometry can be used to calculate the position from the shore to a ship?

The person on the shore can find the angle between the shore and the ship and the ship's captain can find the angle from the ship to shore.  It looks a bit like a pirate ship, so best we can locate their exact position in case we need help!

The sine rule is used to calculate angles in a non-right angled triangle.  The formula is:

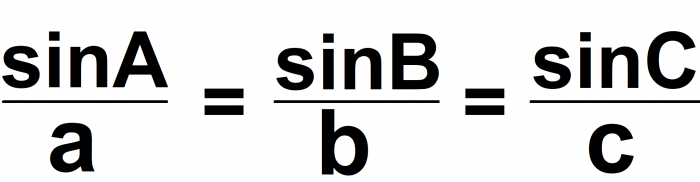
It looks the same as the sine rule for finding a side length. The difference is that the terms have been turned upside down with the angles (sin) being on the top.

You will need a scientific calculator in order to get the sin^-1 button. Make sure it is set to degrees.

Example 1

Calculate angle x.

Step 1: Label the angles of the triangle if it hasn't already been labelled. Our angles are A, B, and C. You are free to pick the order they go in.

Step 2: Label the lengths. This is where you have to be very precise, otherwise the formula will not work. The length opposite angle A must be called a, the length opposite angle B must be called b, and the length opposite angle C must be called c.

A = 60°
B = x
C = (blank)

a = 7cm
b = 6cm
c = (blank)

Step 3: Write the formula that you will need. Notice that length c and angle C are both blank, so in this case, we can leave them out:  

sin A / a = sin B / b

Step 4: Substitute your known values:

sin 60 / 7 = sin x / 6

Step 5: Rearrange the formula to make sin x the subject:

sin x = 6 x (sin 60 / 7)

sin x =  0.74 ...

Step 6: Use inverse sin ("sin^-1") to find the angle. (Just remember to press "shift" + "sin" on your calculator to make it appear).

x = sin^-1 (0.74...)

x = 47.9° (to 3 significant figures)

NOTE: If you try to put it all into the calculator at once, the rules of BIDMAS aren't followed and your answer will be incorrect.

Let's give it a try...