Evaluate Fractional Indices

In this worksheet, students will learn to evaluate integers and fractions with a fractional index number.

Key stage: KS 4

Year: GCSE

GCSE Subjects: Maths

GCSE Boards: Pearson Edexcel, OCR, Eduqas, AQA,

Curriculum topic: Number, Indices and Surds

Curriculum subtopic: Structure and Calculation Powers and Roots

Difficulty level:

---- OR ---- Preview Activity

Worksheet Overview

You may already feel confident with positive and negative index numbers, but we also need to be able to calculate powers represented as fractions.

If you are not feeling 100% about calculating positive and negative indices (e.g 3⁴ or 5^-2), it will be worthwhile revising these topics before trying this more challenging topic.

We need to be able to work with index numbers that are fractions. e.g. 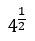or

If we look at the fractional indices in this table, we should spot patterns that will help us work out these values:

e.g. 16²	256	The next step will be to divide our power by 2 (2 divided by 2 = 1). The next power we use will be 1.
16¹	16	256 has been divided by 16, or we could say that we have found the square root of 256. (The next step will divide the power by 2 again. 1 divided by 2 is ½.)
16^½	4	16 has been divided by 4, or we could say that we have found the square root of 16. (The next step will again divide the power by 2 and ½ divided by 2 is ¼)
16^¼	2	4 has been divided by 2, or we could say that we have found the square root of 4.

So 16^½ means we have found the square root of 16 (i.e. the number that multiplied by itself will give us 16).

Let's look at another example now.

means that we need to find the cube root of 27.

In simpler terms, we need to find the number that multiplied by itself 3 times gives 27.

The answer to this is 3.

This could be written as:

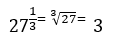
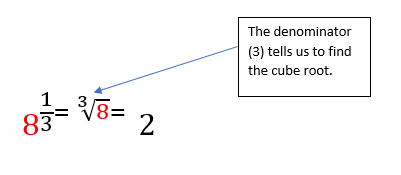
Here's one more example to check you've really got the hang of this.

Let's try some questions now to put this into practise!

You may want to have a pen and paper so that you can compare your working to our explanations written by a teacher.

---- OR ----

Preview activity

What is EdPlace?

We're your National Curriculum aligned online education content provider helping each child succeed in English, maths and science from year 1 to GCSE. With an EdPlace account you’ll be able to track and measure progress, helping each child achieve their best. We build confidence and attainment by personalising each child’s learning at a level that suits them.

Get started